The fact that the battery is disconnected at the end is irrelevant. The voltage across the capacitor is determined by the voltage applied to it. Therefore, it is the same regardless of whether or not there is a dielectric in the capacitor. Therefore, answers (A) and (B) are incorrect. The electric field in the capacitor is determined by the voltage across the capacitor and the distance between the plates. Therefore, it also does not change when a dielectric is inserted between the plates, as long as the plates themselves are not moved. Therefore, answer (D) is false. The effect of inserting a dielectric between the plates of a capacitor is to multiply the capacitance of the capacitor by the relative permittivity (AKA the dielectric constant) of the dielectric material. The relative permittivity (AKA the dielectric constant) is always greater than or equal to one,
therefore, the capacitance of a capacitor increases when a dielectric is inserted. The capacitance is defined as  . The capacitance is increased and the voltage . The capacitance is increased and the voltage 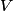 is held constant, therefore is held constant, therefore 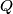 (the charge on the positive plate) must increase. Therefore, answer(C) is wrong. (the charge on the positive plate) must increase. Therefore, answer(C) is wrong. The relationship between the electric field vector and the displacement vector in dielectrics is 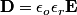 where 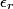 is the relative permittivity (AKA the dielectric constant). Initially, is the relative permittivity (AKA the dielectric constant). Initially, 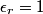 , but after the dielectric is inserted, , but after the dielectric is inserted, 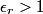 . As discussed above, . As discussed above, 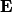 is held constant. Therefore, is held constant. Therefore, 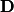 must increase when the dielectric is inserted. Therefore, answer (E) is correct. must increase when the dielectric is inserted. Therefore, answer (E) is correct. |